Prize Winner, Part 1

Home > Prize Winner, Part 1 > Page 3

Andrew thinks the game is too easy, and suggests ways to make the game more challenging. Justyce says that there should be three levels of prizes: small, medium and large, with new rules for winning. The group agrees.

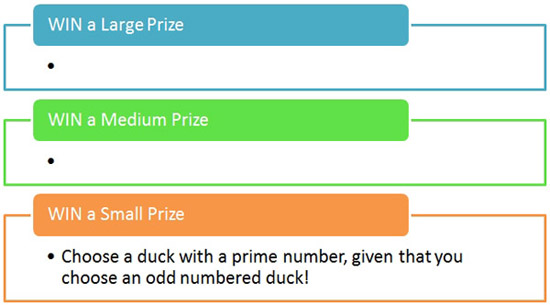
Khalid starts designing a “Rules of the Game” sign. On it, he writes the following:

Real-Life Scenarios

> Text version for animation

Check Your Understanding

Check Your Understanding

You may be wondering what the difference is between having the rule for a small prize state:

1. “What is the probability of a person selecting a prime and odd number?”
   
   versus:
   
   
2. “What is the probabilty of a person selecting a prime number, given that they selected an odd number?”

You already know the answer to (a) is${\displaystyle \frac{9}{30}=\frac{3}{10}}$. This probability is referring to all of the numbers that are odd and prime out of all thirty numbers. In the Venn Diagram, these are the nine numbers that appear in the center out of all thirty numbers.

You also already know from Andrew’s explanation, the answer to (b) is ${\displaystyle \frac{3}{5}}$. We can also see this in the Venn Diagram. Given that the gamer chooses an odd number first, we are limited to the 15 odd numbers. From this circle, we choose the prime numbers. Thus, we get 9 prime numbers out of the 15 odd numbers, or ${\displaystyle \frac{9}{15}}$. This simplifies to the ${\displaystyle \frac{3}{5}}$ Andrew got.

 

 

< Previous Next >