Simply Surveying
Resources for this lesson:
You will use your Algebra II Journal 
on this page. Note that you will need a printed copy of the journal reflection pages to complete the activity.
> Glossary
> Calculator Resources
> Teacher Resources: Instructional Notes
It did not snow as much as the girls had hoped so they had to go to school the following day. They decide to share their surveying work with their teacher. As they are talking, Khalid enters the classroom.
Khalid: Why does the sample data have to be a simple random sample? Why can't you just pick the first ten states, or the first fifteen tsunamis? Why does it have to be random?
Khalid is asking some important questions. Let’s complete the following activity to find out why samples need to be random.
Tsunami Mathematical Task
Tsunamis can be damaging and devastating. They have the power to wipe out entire towns with their powerful waves. However, not all parts of a tsunami are bad. As the large waves rush in, they carry strange and wonderful creatures from the deep ocean. Some of these creatures are well known, while others are new discoveries for scientists.
One of the many creatures washed up by a tsunami is the "purple tsunami crab" (Insulamon palawanense). While this creature is not a new discovery, it does worry scientists. These creatures from the deep pose a possible threat to native species, by either killing them or competing against them for food. Thus, scientists want to study these creatures. To learn more about the "purple tsunami crab," visit the link below:
> Pretty in Purple
(from National Geographic Daily News)
Algebra II Journal: Reflection 3
Pictured here is a colony of fifty "purple tsunami crabs."
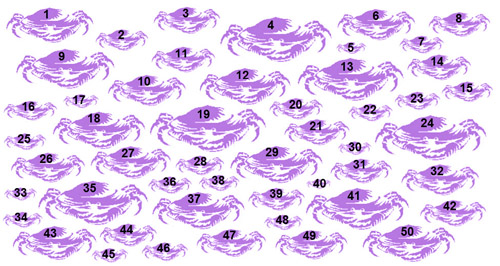
An illustration of these crabs is in your Algebra II Journal . Each crab can be identified by the number that appears on its shell. The table below shows the length of each crab. Note that a crab is measured by the length of its top shell, excluding the legs.
Crab Number |
Shell Length |
Crab Number |
Shell Length |
|---|---|---|---|
1 |
30 |
26 |
27 |
2 |
19 |
27 |
29 |
3 |
24 |
28 |
18 |
4 |
39 |
29 |
33 |
5 |
10 |
30 |
12 |
6 |
27 |
31 |
22 |
7 |
15 |
32 |
28 |
8 |
22 |
33 |
11 |
9 |
39 |
34 |
14 |
10 |
28 |
35 |
40 |
11 |
23 |
36 |
16 |
12 |
32 |
37 |
33 |
13 |
38 |
38 |
15 |
14 |
22 |
39 |
20 |
15 |
17 |
40 |
9 |
16 |
19 |
41 |
37 |
17 |
13 |
42 |
24 |
18 |
33 |
43 |
30 |
19 |
42 |
44 |
20 |
20 |
19 |
45 |
12 |
21 |
23 |
46 |
17 |
22 |
16 |
47 |
25 |
23 |
16 |
48 |
13 |
24 |
40 |
49 |
26 |
25 |
14 |
50 |
33 |
Select 15 crabs from the table above. Record the data for each of the crabs you chose in the table in your journal. A copy of the table is shown below.
Crab Number |
Shell Length |
|---|---|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
MEAN = |
|
Next, make a dot plot of your data. You may create your dot plot using the number line provided in your journal, using your graphic calculator or the Shodor applet . (See the Calculator Resources for instructions about creating a histogram as an alternative to creating a dot plot.)
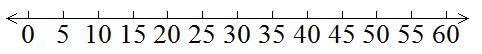
Next, select 15 crabs again using the following process for selecting the crabs.
- Randomly generate 15 numbers using either your graphing calculator (for directions go to http://mathbits.com/MathBits/TISection/Statistics1/Random.htm ) or another random number generator (such as http://www.randomnumbergenerator.com/ ).
- Record the data for each crab in your journal. A copy of the table is shown below.
Crab Number
Shell Length
(millimeters)MEAN=
Make a dot plot of your data. You may create your dot plot using the number line provided in your journal, using your graphic calculator or the Shodor applet . (See the Calculator Resources for instructions about creating a histogram as an alternative to creating a dot plot.)
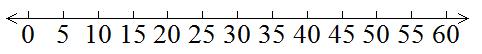
Now respond to the following reflection questions in your journal and submit to your teacher.
- Describe the shape, center and spread of the distribution of sample mean from the first dot plot you made. This was the dot plot where you selected any crabs you wanted.
- Describe the shape, center and spread of the distribution of sample mean from the second dot plot you made. This was the dot plot where your selection of crabs was randomly selected.
- Describe the key differences between the two distributions of sample means.
- Decide which sampling method you think is the better one for estimating the population mean by a sample mean, and explain your reasoning.
